Mathematics is an interesting subject that helps us to solve problems and understand the world better. In math, there's something important called integration. It's used in different areas like physics, engineering, and economics to help us understand and solve problems.

While integration is a powerful tool, some integrals can be quite challenging to solve. That's where advanced methods come into play.

In this article, we'll look at some advanced methods like partial fractions, trigonometric substitutions, and other ways to simplify difficult integrals. These techniques can help us solve complex problems more easily.

Partial Fractions :

Now, let's begin with partial fractions, a method that helps us break down complex fractions into easier ones. This technique makes it simpler for us to work with and understand these fractions.

When faced with a rational function (a fraction with polynomials in the numerator and denominator), we can use partial fractions to express it as a sum of smaller fractions. By doing so, we transform the integral into a more manageable form.

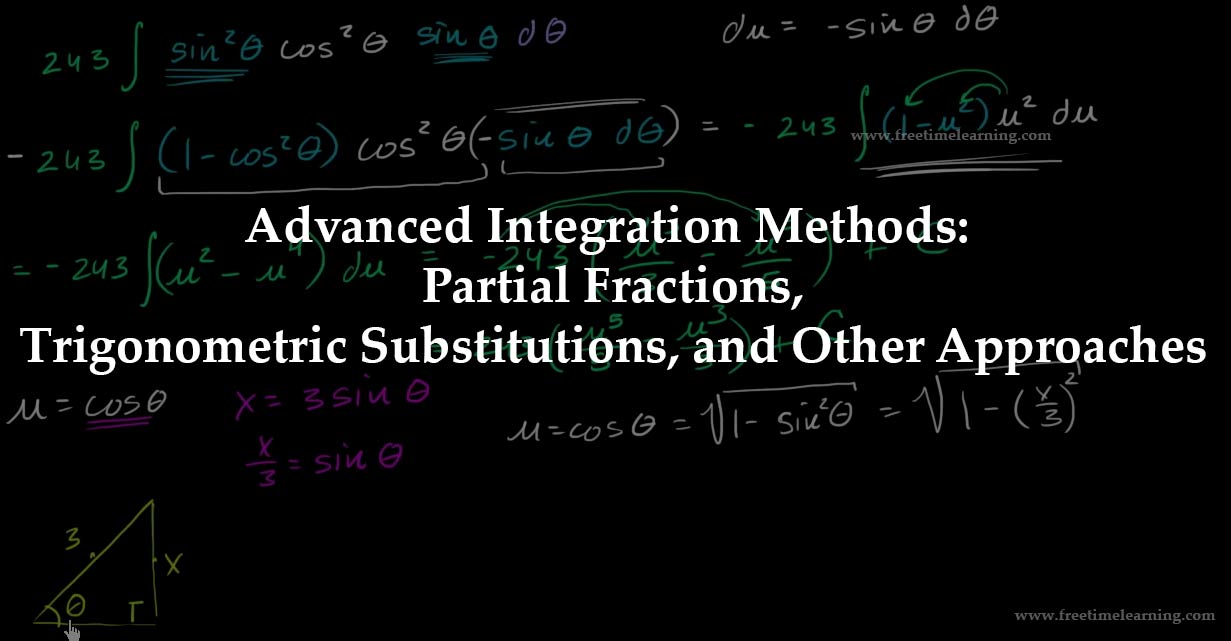
suppose we have the integral  ∫(3x^2 + 2x + 1) / (x^3 + 4x^2 + 5x) dx

Using partial fractions, we can decompose the fraction into its individual components and rewrite the integral in terms of these simpler fractions. This allows us to solve the integral more easily.

Trigonometric Substitutions :

Trigonometric substitutions are handy tools when dealing with integrals that involve square roots or complicated expressions. By replacing a variable with a trigonometric function, we can make the integral easier to solve by using trigonometric relationships or rules.

This substitution simplifies the integral and makes it more manageable for us to work with. We can also calculate the trigonometric substitutions by using the online Trigonometric Substitution Calculator.

There are three common trigonometric substitutions :

a) Substitution 1: x = a * sinθ : This substitution is useful when encountering square roots of the form √(a^2 - x^2). By substituting x = a * sinθ, we can rewrite the integral in terms of trigonometric functions, which makes it easier to solve.

b) Substitution 2: x = a * tanθ : Use this substitution for integrals that involve terms like √(x^2 + a^2). By substituting x = a * tanθ, we can simplify the integral and express it in terms of trigonometric functions.

c) Substitution 3: x = a * secθ : When integrating expressions with terms like √(x^2 - a^2), we can employ this substitution. By substituting x = a * secθ, we can rewrite the integral using trigonometric functions, making it more manageable.

Let's look at an example to demonstrate how powerful trigonometric substitutions can be:

Suppose we have the integral ∫(x^2) / √(9 - x^2) dx.

By substituting x = 3 * sinθ, we can transform the integral into a trigonometric expression. This simplification helps us solve the integral with ease.

Other Advanced Integration Approaches :

Apart from partial fractions and trigonometric substitutions, there are additional techniques that can help us simplify integrals.

a) Integration by Parts : Integration by parts is useful when we need to integrate the product of two functions. It involves using a formula to transform the original integral into a simpler one, which is often easier to solve.

For example, if we have the integral ∫(x * cos(x)) dx, integration by parts provides a systematic way to approach it. We can also use an integral by parts calculator for calculating it online.

b) Improper Integrals : Sometimes, integrals have infinite intervals or include singular points within the interval. These are called improper integrals. Evaluating them requires understanding limits, which help us handle these special cases.

c) Integration of Rational Functions with Square Roots : Integrating rational functions that contain square roots can be challenging. Don't worry! With some smart math tricks like changing the order of equations or using special functions, we can make these integrals easier to solve and understand.

d) Integration of Exponential and Logarithmic Functions : Integrating functions that involve exponential and logarithmic terms often requires specific techniques. Methods like u-substitution or integration by parts allow us to simplify the integrals and find their solutions.

Conclusion :

Advanced integration methods, including partial fractions, trigonometric substitutions, integration by parts, and other approaches, provide us with powerful tools to simplify the process of solving complex integrals. These techniques help us break down complex problems into smaller and simpler parts. This makes it easier for us to solve the problems step by step and find the solutions.

With regular practice and exploration of further applications, you can enhance your proficiency in these advanced integration methods. Remember to approach problems step by step, utilizing the appropriate method for each situation.

By dedicating time and effort to mastering these techniques, you will develop a strong foundation in advanced integration and become more proficient in solving complex integrals.


Q1: Why do we need advanced integration methods?

A : Advanced integration methods are needed to simplify and solve complex integrals that cannot be easily evaluated using basic integration techniques. These methods allow us to solve challenging mathematical problems. Calculate advanced integration methods by online integral calculator.


Q2: How can partial fractions help in integration?

A : Partial fractions help us break down complicated fractions into simpler ones. This decomposition allows us to express the integral as a sum of these simpler fractions, making it easier to solve.

Q3: When should I use trigonometric substitutions?

A : Trigonometric substitutions are particularly useful when dealing with integrals involving square roots or complex expressions. When we replace the variable with a trigonometric function, we can simplify the integral using special trigonometric rules. This makes the integral easier to work with and solve.


Q4: What are some common trigonometric substitutions?

A : There are three common trigonometric substitutions: x = a * sinθ, x = a * tanθ, and x = a * secθ. These substitutions are used in different scenarios to simplify integrals and express them in terms of trigonometric functions.


Q5: Can you explain integration by parts?

A : Integration by parts is a technique used to integrate the product of two functions. It involves applying a specific formula to transform the original integral into a simpler one. This method is helpful in solving integrals that involve products of functions.


Q6: What are improper integrals?

A : Improper integrals are integrals with infinite intervals or singular points within the interval of integration. Evaluating these integrals requires the concept of limits, which allows us to handle these special cases.

Q7: How can I simplify integrals involving rational functions with square roots?

A : Integrating rational functions with square roots can be challenging. However, by employing suitable algebraic manipulations or trigonometric substitutions, we can simplify these integrals and make them more manageable.


Q8: How do I integrate functions involving exponential and logarithmic terms?

A : Integrating functions that involve exponential and logarithmic terms often requires specific techniques, such as u-substitution or integration by parts. These methods allow us to simplify the integrals and find their solutions.

Remember, practice and familiarity with these advanced integration methods will help you become more comfortable and proficient in solving complex integrals.